Analytical Approaches to World Musics
ISSN 2158-5296
Morris
AAWM Journal 11/1 (2023)
Recent Developments Coordinating Melody and Harmony[1]
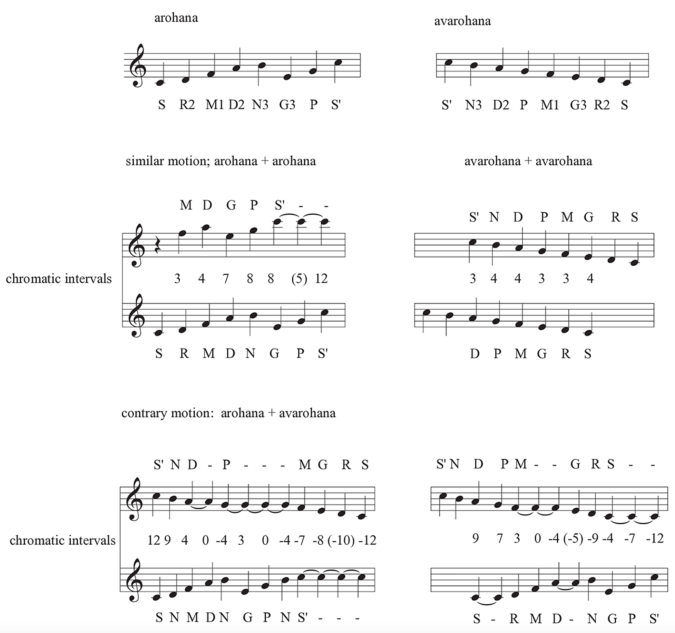
Robert Morris
Carnatic music, Karnatak music, Raga scale harmonization, melakarta, melharmony, theory of Indian classical music
For some time now, both Carnatic and Hindustani musicians have begun to harmonize traditional and classical compositions and implement forms of harmony in improvisation. Nevertheless, the musical results have not been altogether satisfying because important syntactic aspects of both Western and Indian music have been compromised in such endeavors. This paper offers some ways the melodic integrity of Indian music and the harmonic/voice-leading features of Western music can support each other rather than clash.
The paper constructs two-voice frameworks by superimposing an ascending raga scale (ārōhaṇa) on a modal transposition of itself or upon its descending scale (avarōhaṇa). An added proviso is that the vertical chromatic intervals among corresponding notes in each framework must form one of the following chromatic intervals: 0, 3, 4, 7, 8, 9. It is shown that the cardinality of a scale is an important determinant of the possibility of constructing networks. The network format is generalized into interval matrices to cover cases where a raga scale’s arohana and avarohana are highly dissimilar. The paper ends by presenting several methods by which the frameworks can implement the harmonic arrangement of traditional compositions or the composition of new ones.
In sum, just as each raga scale has a number of distinct melodic characteristics (lakshanas), it also has a number of harmonic/voice-leading features that distinguish it from other raga scales.
Robert Morris is Professor of composition and affiliate of the theory department at the Eastman School Of Music, University of Rochester.
[1] For some time now, both Indian and non-Indian composers and performers have harmonized Indian rāgas with chords from western music in genres from Indian cinema music to improvised “fusion” or “world beat.” More recently, both Carnatic and Hindustani musicians have begun to harmonize traditional and classical compositions and implement forms of harmony in improvisation. Sangita Kalanidhi Chitravina N Ravikiran has refined and extended the scope of such ventures with his concept of melharmony that considers how the melodic and harmonic grammars of different musics may effectively and aesthetically interact; for instance, how harmonic treatment may support but not interfere with the melodic aspects of a music. In the case of combining Indian and Western music, the musical grammar of recent western musics, especially jazz, is based on the concept that melodies are determined by harmony—that is, a series of chords (simultaneous notes) determines what notes may occur in a melody as it progresses in time. But if Indian music is to have harmony, the melodies based on rāgas will have to guide the harmony, which will have to be based on the notes in the rāga. The wealth of different rāgas makes this project quite context sensitive; however, the research I will present today helps generalize the harmonization of various rāgas. [2] In 2006, Chitravina N Ravikiran and I published a paper that surveys some of the technical possibilities of melharmony in Carnatic music. The present paper develops these possibilities by studying the properties of Indian and other scales that permit the construction of two-voice polyphonic frameworks. Similar frameworks, often called schemata, have been used in western common practice music as well as jazz to provide a polyphonic base for the harmonization of melodic lines; our two-voice frameworks can similarly function to enable the harmonization of melodies based on different rāgas.[2] [3] Rāgas are complex, context-sensitive pitch-systems that are best described as various networks of notes.[3] An important rāga attribute is its underlying scale. Scale can be defined in two ways. First is the unordered content of a rāga’s notes but written in ascending order. The 72 mēlakarta scales and their janya subsets provide some norms for the notes used in actual ragas.[4] Second, a given rāga determines a unique ascending and descending scale. [4] Nevertheless, the complex ornamentation of some, especially traditional Carnatic, rāgas do not make them susceptible to any kind of harmonization. In Hindustani music, rāgas with subtle shades of intonation and slow gliding ornamentation also make them difficult to harmonize. But there many important rāgas with more stable, relatively unornamented notes, and these can be harmonized effectively using two-voice frameworks.[5]
Basic Terminology
[5] To begin, it is necessary to define a few fundamental terms. The first term is similar motion, (abbreviated SM) this is when two melodies are sung or sounded at the same time and they both ascend or both descend. Second is contrary motion, or CM, where one melody ascends while the other descends. [6] The third term is chromatic interval. When we write the 16 svarasthānas in a circle as below (Figure 1), we see them grouped into twelve positions around the circle.
Figure 1. The 16 svarasthānas.

Example 1. Two frameworks based on mēla Hēmavati.

Example 2. Two frameworks based on the scale of raga Srīrañjani.
[10] Note that the framework in Example 1 formed by similar motion fulfills VC, while the contrary motion framework does not. [11] The reverse situation is found in Example 2; the notes are those of the six-note scale of the rāga Srīrañjani, a subset of mēla 22, Kharaharapriya. Now the contrary motion framework fulfills VC but similar motion does not. Example 3 shows a five-note scale (from the rāga Nāgasvaravāḷi) that achieves VC in two different ways under contrary motion.
Example 3. Two distinct similar motion frameworks based on the scale of raga Nāgasvaravāḷi.
[12] The purpose then of this essay is to provide methods to generate 2-voice frameworks that satisfy VC for as many of the 72 mēlas and their subsets as possible. In addition, we will also develop frameworks for rāgas with vakra melodic motion. [13] A few more technical definitions are needed to advance the next discussions.The adjacent intervals of scales: i-series
[14] A scale can be represented by a series of numbers that add up to 12. Each number stands for the chromatic interval between adjacent intervals in the scale. We call this an i-series.
Figure 2. The i-series of mēla Kīravāṇi.
[15] As shown in Figure 2, the mēla Kīravāṇi has the i-series 2122131.Scales and Modal Equivalence
[16] Scales are cycles of notes where one note is Sa, the “tonic” or starting note. By convention, we write the scale in western notation in ascent starting with Sa set to C-natural. A mode is a cyclic permutation of a scale, so the tonic changes to another note in the scale. Mūrchhana and graha bhēdam are the Indian terms for cyclic permutation. For instance, as illustrated in Figure 3, given the mēla 16, Māyāmāḷavagauḷa, if we take Ma as the Sa of this mēla and change all the svara names accordingly, we have a mode, the mēla 57, Simhēndramadhyamam. A scale and its modes are said to be modal equivalents. So mēla 16 and 57 are modal equivalents.[10] We can determine if two scales are modal equivalents by looking at their i-series; if so, the two i-series will be related under cyclic-permutation or rotation.
Figure 3. The modal equivalents Māyāmāḷavagauḷa and Simhēndramadhyamam.
Inversion
[17] Inversion is a specific operation on a scale; it turns it upside down, so to speak. As the chart in Figure 4 shows, inversion changes S to S, R1 to N3, R2 to N2, G1 to D3, and so forth to M2 remaining the same.
| C | S | becomes | S | C |
|---|---|---|---|---|
| Df | R1 | ‘’ | N3 | B |
| D | R2 | ‘’ | N2 | Bf |
| D# | R3 | ‘’ | N1 | Bff |
| Eff | G1 | ‘’ | D3 | A# |
| Ef | G2 | ‘’ | D2 | A |
| E | G3 | ‘’ | D1 | Af |
| F | M1 | ‘’ | P | G |
| F# | M2 | ‘’ | M2 | F# |
| G | P | ‘’ | M1 | F |
| Af | D1 | ‘’ | G3 | E |
| A | D2 | ‘’ | G2 | Ef |
| A# | D3 | ‘’ | G1 | Eff |
| Bff | N1 | ‘’ | R3 | D# |
| Bf | N2 | ‘’ | R2 | D |
| B | N3 | ‘’ | R1 | Df |
Figure 4. Inversion of svaras.
[18] Three examples of inversion are given in Figure 5.
Figure 5. Examples of inversion.
[19] Under inversion, mēla 29 changes into mēla 8 and rāga Mōhanam changes into rāga Hindōḷam. Some mēlas and/or rāgas may not change into scales that are mēlas or rāgas. For instance, Mēchakalyāṇi mēla 65 does not change into a mēla under inversion; the inversion has two Mas and no Pa. As also shown, the inversion of a scale transforms the numbers in an i-series into their negatives.
Example 4. Transformations on mēla 21, Kīravāṇi.
[20] We may combine inversion with retrograde and/or cyclic permutation In Example 4, mēla 21, Kīravāṇi is used to show this. These transformations permit an important invariance: if a scale can generate any of the kinds of frameworks discussed in the rest of this paper, then the inversion, and/or the retrograde and/or the cyclic permutation of that scale will also be able to generate a framework of the same kind.Constructing Similar Motion Frameworks
[21] The requirement for a scale producing a two-voice framework under similar motion satisfying VC is that each pair of adjacent intervals in the scale’s i-series adds up to 3 or 4. This guarantees that intervals between the simultaneous notes will be 3, 4, 8, or 9 chromatic intervals apart. This is because notes two (or 6) positions apart will occur simultaneously when the superimposed scale is shifted by 2 (or 6) steps.
Example 5. SM frameworks with mēla 26, Chārukēśi.
[22] Example 5 slows mēla 26, Chārukēśi with the i-series 2212122; each pair of adjacent intervals sums to 3 or 4. Those are the intervals permitted in the VC. Under shifting by two or six steps, notes two positions in the scale apart will occur as simultaneities in the framework. [23] 17 mēlas have this property. These are called all-third mēlas.[11] [12] [24] Other scales and mēlas can also form frameworks under SM, but in these cases some of the vertical intervals are not members of the VC; these are called incomplete frameworks. In particular, we examine cases where only one of the simultaneous intervals is not from the VC. [25] Example 6 shows an incomplete framework using mēla 46. The outlier interval is 5. The framework projects members of VC providing one omits the Df (R1) in the top voice. There are two other modal-equivalent mēlas that have this property.[13]

Example 6. Incomplete SM network for mēla 46, Sadvidhamārgini.

Example 7. Four incomplete SM frameworks.
[26] The next four incomplete frameworks in Example 7 would have an interval 2 among their verticals. This is avoided in three of the four cases by sustaining a note into the omitted notes place. Thus, the SM framework for these mēlas is complete, even if one of the notes in top voice is omitted. (The last of the examples, using mēla 72, simply omits a note.)
Example 8. Maximally incomplete SM framework for mēla 39, Jhālavarāḷi.
[27] A contrast is mēla 39, Jhālavarāḷi, in Example 8 whose framework contains only two members of VC, both interval 4. Thus, it produces a maximally incomplete SM framework. Varāḷi, the well-known chromatic rāga, can be derived from this mēla. [28] Table 1 shows the mēla scales that produce complete (the all-third mēlas) and minimally incomplete SM frameworks. This is 36 mēlas, half of the number of mēla scales, many of them highly chromatic.
Table 1. Mēla scales that produce complete and minimally incomplete SM frameworks.
Constructing Contrary Motion Frameworks: Six-tone Scales
[29] No seven-tone scale can produce complete contrary motion (CM) frameworks. On the other hand, many six-tone scales have this property. The way to understand this is to consider the six-tone scale to be a subset of a 7-tone scale, in this case, a mēla. [30] Given a mēla with ascending notes (a b c d e f g), we extract one note, in this case d. If the intervals between a and c and between e and g are members of the VC, then we can align the six-tone scale with its shifted retrograde to make an upper and lower voice combination. This is demonstrated in Figure 6. The notes of the mēla are a, b, c, d, e, f, and g, in ascending order. Note d is extracted and the forward and retrograde scales are aligned around the extracted tone d. This configuration of notes is shown here. [31] The extracted note d is the axis of alignment (but is not used in the 6-note scale). The chromatic intervals between a and c is x and x’, and intervals between e and g is y or y’. If and x and y are 3, 4, 8, or 9, the framework has VC.
Figure 6. Aligning a six-tone scale with its shifted retrograde to make an upper and lower voice combination.

Example 9. Six-note CM frameworks from 7-tone scales.
[32] The top portion of Example 9 is a specific example of a CM framework derived from mēla 22, an all-third mēla. By contrast, the bottom part of Example 9 shows a CM framework using a mēla, nevertheless, whose i-series shows three thirds of 2 and 5 semitones. [33] But despite the fecundity of six-note scales that can generate CM frameworks, most of them are not traditional rāgas in their own right. In fact, there are only a few 6-tone well-known rāgas whose scales are subsets of a mēla. One of them is displayed in Example 10.
Example 10. A CM framework based on a 6-tone raga scale.
CM Frameworks: Five-tone Scales
[34] We now turn to frameworks derived from 5-tone scales. While there are no SM frameworks, there are some CM frameworks. [35] We can show the seven-tone scale as a circle of seven notes in Example 11.
Example 11. A seven-tone ascending scale written as a cycle.

Example 12. Two adjacent tones are extracted from a seven-tone scale.

Example 13. Species 1 5-tone scale.
[36] From a seven-tone scale, we may derive five-tone scales by extracting two notes. This can be done in three ways producing three species of 5-tone scales. [37] Species 1: we subtract 2 adjacent tones as illustrated in Example 12. [38] This leaves a gap of three steps in the resulting scale. There are only a few rāgas that use this species of 5-tone scales. One of them is given in Example 13.
Example 14. Two tones one step apart are extracted from a seven-tone scale.
[39] Species 2: In Example 14 we select 2 tones that are separated by a single tone and extract the former.
[40] There are a quite a number of rāgas whose scales are of this species. Example 15 displays two of them.
Example 15. Species 2 5-tone scales.

Example 16. Two tones two steps apart are extracted from a seven-tone scale.

Example 17. Species 3 5-tone scales.
[41] Species 3: We extract 2 tones that are separated by two tones. See Example 16.[14] [42] There are quite a few rāgas that are included in this species. For instance: Mōhanam and Śuddha dhanyāsi are presented in Example 17. [43] Double CM frameworks are available with some 5-tone scales of species two. On the top of Example 18, using the scale of rāga Hamsadhvani, we produce a CM framework that satisfies VC. In addition, using a different retrograde shift, we also produce a second CM framework, as on the bottom of Example 18.
Example 18. CM frameworks from species 2 5-tone scales.
CM Frameworks for Scales with Less Than 5 Tones
[44] Scales of less that 5-tones that permit VC are only of a few types:Two 3rds connected by a 2nd (seventh-chord)
Consecutive 3rds (triad)
Consecutive steps (three adjacent notes)
Consecutive steps (three adjacent notes) connected to a 4th interval. [45] These scale fragments are useful in producing frameworks from rāgas with vakra motion. [46] Example 19 provides eight scale extractions resulting in four-tone scales for mēla 21 Kīravāṇi.

Example 19. Worked examples of the eight cases of extraction on the mēla 21, Kīravāṇi.
Incomplete CM Frameworks
[47] As we pointed out before, 7-note scales or mēlas may produce a minimally incomplete CM framework. We simply don’t extract the note that would produce a 6-tone CM framework. The non-extracted tone does not produce a VC interval in the framework, but it can be used as a passing tone. Two incomplete CM frameworks are shown in Example 20; the second is completed by either employing passing tones or sustaining notes.
Example 20. Incomplete CM frameworks.
[48] Other types of incomplete CM frameworks can be built from the bottom up. For instance, given a 4-tone scale—such as C Ef F Af—that can produce a CM framework, we can add a note so that the resulting 5-tone scale (species 3) cannot generate a complete CM framework. In Example 21, however, we can use the added note as a passing tone in the incomplete network.
Example 21. Generating CM incomplete frameworks from the bottom up.
3-voice Frameworks
[49] We have shown that if a scale contains a subset with VC, then the scale can produce frameworks with VC by the use of passing tones. If more than one subset can be found, a three-voice framework can be constructed. See Example 22. The top two systems show two different CM frameworks with passing tones. These are combined to form the three-voice framework below. In the 3-part framework, the top two voices move roughly in SM and are incomplete (having vertical intervals among them that are not from the VC), and there are even parallel fifths. The presence of CM motion between each of the top voices with the bottom voice seems to mitigate these features.
Example 22. Two CM frameworks for the mēla 22, Kharaharapriya, with passing tones based on the embedded 6-note scales C D Ef F G Bf (S R2 G2 M1 P N2) and C D Ef G A Bf (S R2 G2 P D2 N2) and their three-voice combination.
Frameworks for Rāgas with Vakra Movement
[50] Many rāga scales are not merely strictly ascending and descending but have different omitted notes and/or meanders. In order to construct frameworks for such zigzag rāga scales one uses chromatic interval matrices and traces paths on them. [51] Table 2 shows the chromatic interval matrix for the rāga Janarañjani.
Table 2. The chromatic interval matrix for the rāga Janarañjani.
[52] On the matrix the rāga is written in numbers is given along to top (left to right) and side (top to bottom) of the matrix. The ārōhaṇa is followed by the avarōhaṇa. The body of the matrix gives all the chromatic intervals between the notes of the rāga; but on the slide only the intervals in the VC plus 7 are given, all other intervals are omitted. The matrix is divided into four quadrants; the upper left quadrant gives the intervals between the arohana and itself; the lower right quadrant gives the intervals between the avarōhaṇa and itself; the lower left and upper right gives the intervals between the ārōhaṇa and the avarōhaṇa. By tracing paths to the right and/or down on
Example 23a. Frameworks for rāga Janarañjani.
the matrix—moving like a King in chess—one can determine complete or incomplete frameworks for the rāga from which it is derived, in this case Janarañjani.[15] Example 23a shows some frameworks for this rāga.
[53] The next examples give the matrices for rāgas Kadanakuthūhalam and Rītigauḷa, each followed by some frameworks. Note in a few cases, the perfect fourth, the chromatic interval of 5 steps is permitted since it is consonant in Indian music, if not in the West. [54] Table 3 shows the chromatic interval matrix for Kadanakuthūhalam.
Table 3. The chromatic interval matrix for Kadanakuthūhalam.
Example 23b. Frameworks for rāga Kadanakuthūhalam.
[55] Table 4 shows the chromatic interval matrix for Rītigauḷa.
Table 4. The chromatic interval matrix for Rītigauḷa.

Example 23c. Frameworks for rāga Rītigauḷa.
Composing with Frameworks
[56] Melodies based on rāgas (or not) do not solely progress up and down by step. They present various permutations of their scales. In Indian music, however, rāgas prescribe pitch movement according to groups of key phrases called sañcāra or prayōga, many of which are not directly implied by scale of the rāga. Nonetheless, the permutations of a rāga scale will find an accompanying voice in the frameworks that the scale permits.
Example 24. Composing with frameworks.
[57] For instance, in Example 24 consider mēla 23, Gaurimanōhari. This is an all-third scale so it has a SM framework and its various six-tone subsets provide CM frameworks. Three frameworks are presented in Example 24. [58] Now, look at Example 25. If a melody in this rāga presents the following phrase (D Ef G F A C or R G P M D S’), then the frameworks in Example 24 offers three different accompanying 2-voice counterpoints. The bottom voices of each framework are permuted in the same way the top voice is permutated into the melody. Segments selected from frameworks can be mixed in various ways, as demonstrated at the bottom of the example.
Example 25. Accompanying a phrase with the three Gaurimanōhari frameworks.
[59] Thus, the frameworks themselves produce a number of different counterpoints to the same melody, and when mixed, the result may seem to have little connection to any of the frameworks. [60] Now we will construct counterpoints for Bahudāri, a 6-tone janya rāga of the 22nd mēla, Harikhāmbōji. See Example 26. Note that Bahudāri’s descending scale omits a note the ārōhaṇa, A (D2). This presents some complications in constructing frameworks for the rāga. Consequently, both the SM and CM frameworks are incomplete. Moreover, in the incomplete SM framework there are adjustments involving passing tones of Bf (N2) in the ascent and F (M1) in the descent. The CM framework is derived two ways: by a 6-tone scale derived from mēla 22 by extracting the D (R2) and by adding a tone to the subset of the rāga scale (C E G Bf or S G2 P D2); the ascending note A (or Dha) is passing. A counterpoint by mixing the CM and SM frameworks is shown as well.
Example 26. Frameworks for rāga Bahudāri.
[61] Since we are dealing with a rāga, I will present a few of the most salient sañcāra phrases that identify Bahudāri versus other rāgas derived from mēla 22. These are given in Example 27, and a few are harmonized in Example 28. The trick is to make sure the added voice generally follows the successions in the sañcāra; otherwise the added voice will seem only remotely derived from Bahudāri, if at all.
Example 27. Sañcāra for rāga Bahudāri (after Bhagyalekshmy, 2006, 98).

Example 28. Counterpoints for some of the sañcāra of rāga Bahudāri based on mixtures of SM and CM frameworks.
[62] Example 29 displays a portion of a melharmonic arrangement based on the popular composition, “Vātāpi gaṇapatim bhajēham” by Muthuswami Dikshitar in rāga Hamsadhvani. Since this five-tone rāga permits two distinct CM frameworks (as already illustrated in the Example 18), the example harmonizes the last section of the caraṇam in three parts, two playing the melody of the composition in contrary motion.
Example 29. Three-voice harmonization of a caraṇam from “Vātāpi gaṇapatim bhajēham” by Muthuswami Dikshitar.
Summary
[63] In addition to a rāga’s unique melodic attributes, the scale of each rāga has distinct harmonic/polyphonic potentials as presented by two-voice frameworks. However, composition with frameworks is not automatic, one must take into account the melodic characteristics of the rāga to be harmonized. [64] We have shown that complete SM frameworks are primarily associated with 7-tone scales. However only 17 mēlas (the all-third mēlas) have this property. [16] Complete CM frameworks are not available for 7-tones scales. [65] With the exception of two scales, scales of 6 notes or less do not produce complete SM networks. However, many provide possibilities for CM frameworks. [17] [66] CM incomplete frameworks can also be generated for all cardinalities of scales and made complete by the use of sustained or passing tones. [67] As for future research, there are three obvious directions: 1) study frameworks based on other definitions of VC; 2) define the intervals and their sequences permitted in three–or-more-voice frameworks; 3) construct chord sequences and grammars based on the frameworks (perhaps providing the outer voices for chord progressions (as in Western music)).References
Bhagyalekshmy, S. 1990. Ragas in Carnatic Music. Madras: CBC Publications.
Morris, Robert, and Chitravina N. Ravikiran. 2006. “Ravikiran’s Concept of Melharmony: An Inquiry into Harmony in South Indian Rāgas,” Music Theory Spectrum, 28(2): 255–76.
[1]. This article was previously published in The Journal of the Music Academy, Madras Vol. 89 (2018); it is reprinted here with the kind permission of the Music Academy.
[2]. Various frameworks can support various and sundry chord grammars, each associated with different rāgas. But this is outside the scope of this study.
[3]. The Indian term for “note” is svarasthāna.
[4]. Indian music uses the term janya to indicate that a scale is a subset of a mēla. The terms sampūrna, ṣāḍava, auḍava and svarāntara identify the cardinality of the scale—7, 6, 5, 4 tones, respectively. Rāga scales may be different in ascent from the descent: for instance, 5 tones up and seven tones down (called audava – sampūrna), the ascending and descending scale may not be linear by employing zig-zag contours designated as vakra. Also, the mēla from which the ascending scale is derived is sometimes different from the mēla of the descent.
[5]. Dhrupad musicians such as the Dagar and Gundecha Brothers improvise such simple harmonizations in the later stages of alap. (Ālāp is the free rhythmic, opening section of a typical Hindustani classical performance.)
[6]. These intervals will often be thirds and sixths, but diminished 7ths and 4ths, augmented 5ths and 2nds, are also permitted.
[7]. By omitting 5 and 7, the voices in the framework may cross without complication.
[8]. To be clear, the tuning of intervals in Indian music are based on frequency ratios, and 12-equal tones per octave only approximates such ratios. Moreover, as in western music, there are many candidates for 12-unequal-tones per octave tunings, which are based on frequency ratios. Thus, the use of chromatic intervals is an approximation of the actual intervals used in the performance of Indian music. We will only assume that the intervals used in the music are acoustically pure and “pleasant to the ear” to both the Indian and western musician.
[9]. The VC might include perfect fourths, minor sevenths, for instance. Different scales from the ones we discuss in this study will satisfy other definitions of VC. Of course, the VC can be defined differently to connect a rāga with various genres of western music, such as jazz, or post-tonal music.
[10]. Not all the modal equivalents of a mēla are also mēlas. This is because the fifth step (Pa) of a mēla music be a perfect fifth (seven chromatic intervals from the tonic (Sa)).
[11]. These scales could also be called all-sixth mēlas.
[12]. Another property: the tertian triads of an all-third mēla are of only the four Western tonal types: major, minor, diminished, and augmented.
[13]. As mentioned above, the series of thirds (separated by one note) in a scale can be read off the i-series by summing successive pairs in the series. So mēla 46 has the i-series 1231212; summing the pairs in the series results in 3543333.
[14]. There are no other species since, for instance, extracting 2 notes that are separated by three tones, is the same as extracting two tones two tones apart (as in species 3).
[15]. This use of matrices is described in “Ravikiran’s Concept…” cited above.
[16]. That is, without repeated or passing tones. The 55 other mēlas can be ranked according to the degree that they incompletely fulfill VC under SM. For instance, six of seven vertical intervals fulfill VC.
[17]. The exact number of 6-tone scales is 249, determined by a computer-assisted search. There are 315 5-tone subsets of the mēlas that can produce CM frameworks.